Mathematician John Conway loved to invent games he could use to explore mathematical relationships. One game he created in the late 1960s became one of the first computer games because it was so easy to code: the Game of Life.
The rules of the game are pretty simple. Starting with an empty rectangular grid of "dead" cells, you turn on as many of them as you like to be "live" in creating an initial pattern. Once you've created that pattern, the rules of the game of life take over. In each turn of the game, a live cell that shares a border with two or three other live cells will survive, or in the case of a dead cell that borders two or three live cells, it will come alive. But if the number of live neighbors for a cell either drops below two or rises above three, it will turn off and die.
What makes the game fascinating to mathematicians is that certain initial settings will create unique patterns as the game progresses. Some may live up to the game's name and become self-replicating. Others may stagnate. Still others enter into an infinitely repeating cycle. This last category are called oscillators.
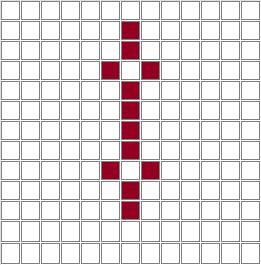
Here are a few examples of the initial patterns for oscillators that will go through a number of cycles in the Game of Life before repeating. We've indicated the number of cycles and the name mathematicians have given to each.
(2) The Blinker:
(8) Figure Eight:
(14) The Tumbler:
(15) Pentadecathlon:
Mathematicians have been playing Conway's Game of Life for over six decades, trying to find patterns that will produce oscillators that go through a minimum of two to an infinite number of cycles. They had mostly succeeded, finding initial patterns that would oscillate between any number of stages before repeating. We say "mostly succeeded" because they hadn't found initial patterns that would go through a period of either 19 or 41 stages.
Until December 2023, when Nico Brown, Carson Cheng, Tanner Jacobi, Maia Karpovich, Matthias Merzenich, David Raucci, and Mitchell Riley posted a preprint paper with their proof that Conway's Game of Life is "omniperiodic", which is the fancy mathematical way of saying you can always find an oscillator that will go through any number of stages you want before they repeat. Including the previously missing cycles of 19 and 41. Quanta's Alex Stone has written a good overview of the paper and how the missing oscillators were found.
We're marking that occasion with a tweak to the version of John Conway's Game of Life we previously posted to accommodate the discovery of an oscillator that will go through 19 stages before repeating (we had sized our version to fit on most mobile phone screens, we needed more rows and columns for this version to accommodate the newly discovered 19-stage oscillator, called "Cribbage"):
If you want to play the Game of Life with this oscillator or any of the others that have been found that will fit on this grid, here you go! If you're accessing this article on a site that republishes our RSS news feed, please click through to our site to play the game on your device.
Click on table cells to toggle the cells as alive or dead.
Click the Start Reproducing button to Start and Stop
We opted out of expanding our grid to accommodate the 41-stage oscillator, known as the "p41 pi-heptomino hassler", because setting up its initial pattern would be quite a hassle on a mobile device.
But that raises a new mathematical question, doesn't it? What is the smallest size rectangular grid that can accommodate a 41-stage oscillator? Or for that matter, the oscillators that go along for any particular number of cycles?
A mathematician's work is never really done, is it?
Image credit: Animated Game of Life Light-Weight Space Ship image by RodrigoCamargo on Wikimedia Commons. Public domain image.
Welcome to the blogosphere's toolchest! Here, unlike other blogs dedicated to analyzing current events, we create easy-to-use, simple tools to do the math related to them so you can get in on the action too! If you would like to learn more about these tools, or if you would like to contribute ideas to develop for this blog, please e-mail us at:
ironman at politicalcalculations
Thanks in advance!
Closing values for previous trading day.
This site is primarily powered by:
CSS Validation
RSS Site Feed
JavaScript
The tools on this site are built using JavaScript. If you would like to learn more, one of the best free resources on the web is available at W3Schools.com.
Other Cool Resources
Blog Roll
