The Navier-Stokes equations describing the flow of fluids are among the most useful math equations that have ever been developed. So much so that if a mathematician should someday demonstrate they can either demonstrate the equations will always work or can provide an example where they do not, they will win a $1 million prize from the Clay Mathematics Institute for solving one of its Millennium Problems.
In doing that, the eventual winner of the prize will be able to predictably explain how order, in the form of the smooth, laminar flow of an incompressible (contant density) fluid, transitions into disorderly turbulence, as illustrated in the following mesmerizing two-and-a-half minute video from the Lutetium Project:
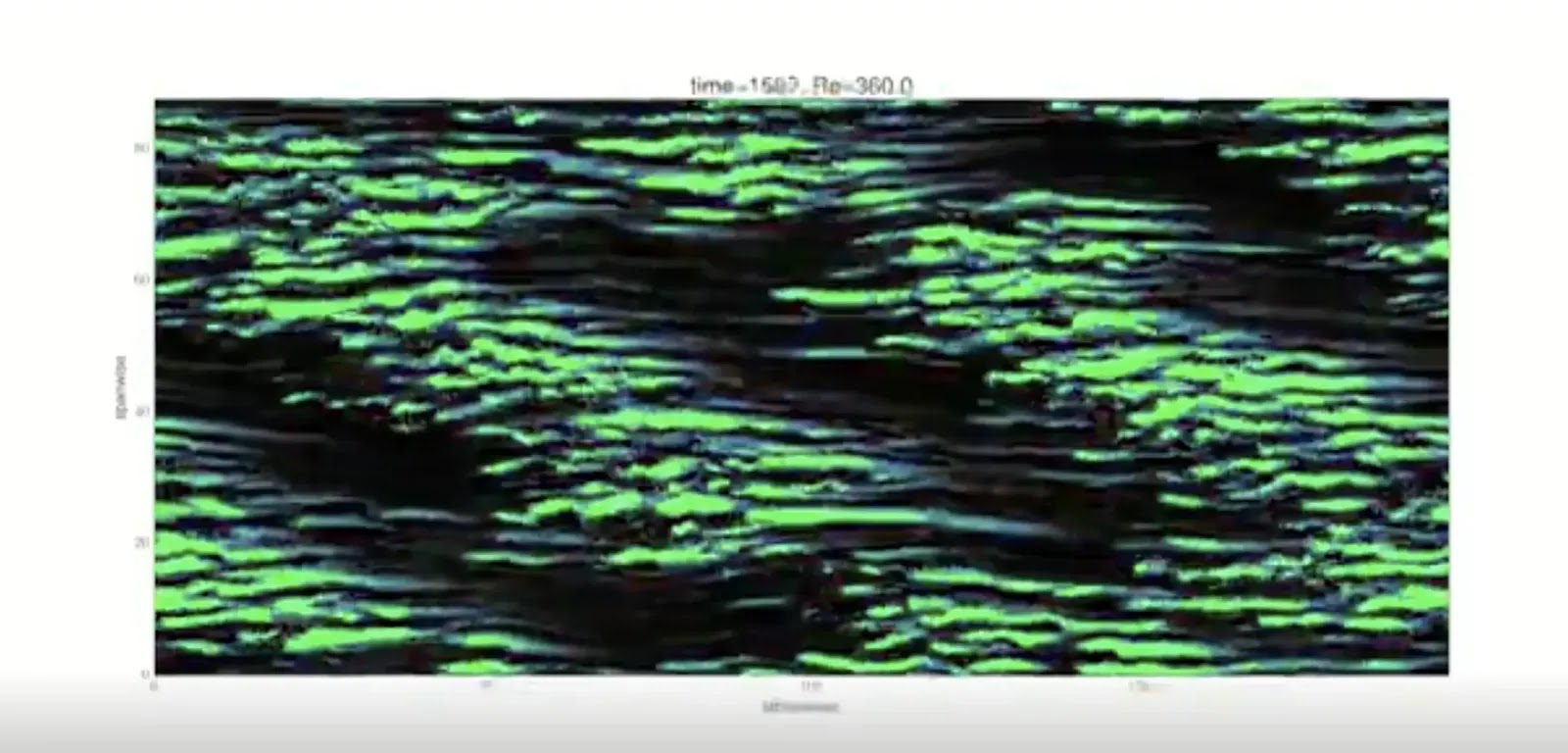
And they will be also be able to accurately describe how some sense of order might spontaneously emerge from turbulent flow, as recently modeled for a recent paper using the Navier-Stokes equations by Florian Reetz, Tobias Kreilos and Tobias M. Schneider of the Ecole polytechnique fédérale de Lausanne (EPFL). The following image from EPFL shows the emergence of an orderly, layered structure within a turbulent flow from their modeling work.
Here's why the application of Navier-Stokes equations to reveal this pattern is such a big deal:
Though physicists had observed this phenomenon experimentally, they can now explain why this happens using fundamental fluid dynamics equations, bringing them a step closer to understanding why particles behave in this way. [The Biggest Unsolved Mysteries in Physics]
In the lab, when a fluid is placed in between two parallel plates that are moving in opposite directions from each other, its flow becomes turbulent. But after a little while, the turbulence starts to smooth out in a striped pattern. What results is a canvas of smooth and turbulent lines running at an angle to the flow (imagine slight wind-created waves in a river).
"You get structure and clear order out of the chaotic motion of turbulence," said senior author Tobias Schneider, an assistant professor in the school of engineering at the Swiss Federal Institute of Technology Lausanne. This "kind of weird and very obscure" behavior has "fascinated scientists for a long, long time."
Physicist Richard Feynman predicted that the explanation must be hidden in fundamental equations of fluid dynamics, called the Navier-Stokes equations.
But these equations are very difficult to solve and analyze, Schneider told Live Science. (Showing that the Navier-Stokes equations even have a smooth solution at every point for a 3D fluid is one of the $1 million Millennium Prize problems.) So up until this point, no one knew how the equations predicted this pattern-forming behaviors. Schneider and his team used a combination of methods, including computer simulations and theoretical calculations to find a set of "very special solutions" to these equations that mathematically describe each step of the transition from chaos to order.
While not the kind of mathematical proof that will win the million-dollar Millennium Prize, Reetz, Kreilos and Schneider have created a remarkable demonstration of the ability of Navier-Stokes equations to accurately describe some of the chaotic mechanics of fluids in motion that are observed in the real world.
As for the status of the Navier-Stokes Millennium Prize, the jury is still out on whether Tristan Buckmaster and Vlad Vicol's initial work demonstrating that the equations do not always generate unique solutions will claim the prize, which we have previously described as the biggest math story of 2017. They have since teamed with Maria Colombo on a new paper (preprint available via arXiv) in following up the topic.
References
Florian Reetz, Tobias Kreilos & Tobias M. Schneider. Exact invariant solution reveals the origin of self-organized oblique turbulent-laminar stripes. Nature Communications 10, 2277 (2019). DOI: 10.1038/s41467-019-10208-x. 23 May 2019.
The Lutetium Project. The transition to turbulence. [Online Video]. 15 November 2016.
Image Credits via Creative Commons Attribution 4.0 International License.
Labels: data visualization, math, physics
Welcome to the blogosphere's toolchest! Here, unlike other blogs dedicated to analyzing current events, we create easy-to-use, simple tools to do the math related to them so you can get in on the action too! If you would like to learn more about these tools, or if you would like to contribute ideas to develop for this blog, please e-mail us at:
ironman at politicalcalculations
Thanks in advance!
Closing values for previous trading day.
This site is primarily powered by:
CSS Validation
RSS Site Feed
JavaScript
The tools on this site are built using JavaScript. If you would like to learn more, one of the best free resources on the web is available at W3Schools.com.