The maths that make modern life possible are often hidden to us. For example, when we use GPS apps to navigate, we don't even notice the math of three-dimensional trilateration being done to make it possible. We just use the app and, almost magically, we end up where we set out to go by following its directions.
It's something of a mistake to say that math is "hidden". With GPS technology, we know there is a lot of maths involved and we know there are electronic computing devices doing them for us. But what about mathematics that has been physically built into our world to make something possible, but which is completely out of sight?
If you ever travel to London, there's a monumental example of where that has been done, in the shape of the dome of St. Paul's Cathedral. Most photographs don't do it justice, but this one gives a true sense of its scale:
The architectural plan for St. Paul's Cathedral's dome was developed by Christopher Wren using the late 1600s' most cutting edge mathematical and engineering insights. His solution to designing the monumental structure of the dome was to incorporate a hidden second dome within the exterior hemispherical dome. This secondary dome would have the cross section of a catenary arch that could fully support its weight. That catenary dome would then be almost fully hidden from sight by an interior hemispherical dome that would become the cathedral's ceiling.
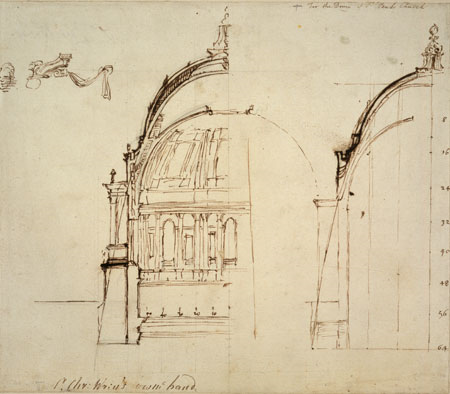
Here's where maths really come into the structure. Wren needed to identify a formula that would approximate the shape of the exterior hemispheric dome that would also approximate the ideal catenary arch cross-sectional shape needed to make the overall structure as strong as possible. Wren's solution was to use the cubic curve given by the formula y=x³ to shape the hidden structure of the catenary dome. Here's a 1690 sketch of Wren's plan for the dome showing the result of his maths:
Plus+ describes why Wren chose this particular cubic formula:
This early sketch (c. 1690) of the triple dome design shows Wren using a mathematical curve to define the shape of the middle dome; the cubic curve y=x³ is clearly plotted on axes marked on the design. The curve not only defines the shape of the middle dome but also the height and width of the surrounding abutments, positioned so as to contain a continuation of the cubic curve to ground level. Wren was applying the theory of his colleague Robert Hooke about the mathematical shapes of ideal masonry domes and arches, one of the earliest instances of mathematical science being used as part of the design process.
In 1675 Hooke published the anagram Ut pendet continuum flexile, sic stabit contiguum rigidum inversum, which translates to "as hangs the flexible line, so but inverted will stand the rigid arch". Hooke had correctly understood that the tension passing through a hanging cord is equivalent to the compression in a standing arch. And so the natural form of a hanging cord — a catenary — would also be the shape of the line of thrust of an arch. For an arch to be stable it needs to contain this line of thrust, either in the material of the arch itself or in its abutments. Therefore the ideal shape for a masonry arch, the shape requiring the least material, is a catenary.
Hooke and Wren thought that the ideal shape of a masonry dome would be the cubico-parabolic conoid created by rotating half of the cubic curve, y=x³. Their mathematical descriptions were very close but the correct equations defining the shape of the catenary and the ideal dome were discovered much later (you can find the details in an excellent paper by Jaques Heyman).
The design of the triple dome continued to evolve after this drawing, with the use of experimental models and the impacts of economics and aesthetics deciding its final shape. The middle dome, as finally constructed, is no longer the pure geometric form of the sketch. But it is clear that its shape is derived from the mathematical concept of a cubic curve, one of the most awe-inspiring instances of the role of mathematics in architecture.
The following public lecture by Gresham College Professor of Geometry Sarah Hart discusses more of Wren's mathematical insights. We've set the video to start when she begins discussing Wren's contributions to architecture at about 35 minutes in:
The full lecture is well worth your time. Wren's mathematical achievements have practical applications far beyond architecture.
Image credit: Photo by Szymon Shields on Unsplash.
Welcome to the blogosphere's toolchest! Here, unlike other blogs dedicated to analyzing current events, we create easy-to-use, simple tools to do the math related to them so you can get in on the action too! If you would like to learn more about these tools, or if you would like to contribute ideas to develop for this blog, please e-mail us at:
ironman at politicalcalculations
Thanks in advance!
Closing values for previous trading day.
This site is primarily powered by:
CSS Validation
RSS Site Feed
JavaScript
The tools on this site are built using JavaScript. If you would like to learn more, one of the best free resources on the web is available at W3Schools.com.